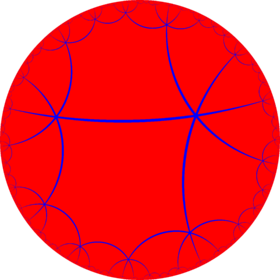
Alternated order-4 hexagonal tiling

{{{U443_0-name}}}
Poincaré disk model of the hyperbolic plane
Type	Hyperbolic uniform tiling
Vertex configuration	(3.4)4
Schläfli symbol	h{6,4} or (3,4,4)
Wythoff symbol	4 | 3 4
Coxeter diagram	or
Symmetry group	[(4,4,3)], (*443)
Dual	Order-4-4-3_t0 dual tiling
Properties	Vertex-transitive

In geometry, the alternated order-4 hexagonal tiling or ditetragonal tritetratrigonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of (3,4,4), h{6,4}, and hr{6,6}.

Uniform constructions

There are four uniform constructions, with some of lower ones which can be seen with two colors of triangles:

*443	3333	*3232	3*22
=	=	= =	=
(4,4,3) = h{6,4}		hr{6,6} = h{6,4} 1⁄2

Related polyhedra and tiling

Uniform tetrahexagonal tilings
Symmetry: [6,4], (*642) (with [6,6] (*662), [(4,3,3)] (*443) , [∞,3,∞] (*3222) index 2 subsymmetries) (And [(∞,3,∞,3)] (*3232) index 4 subsymmetry)
= = =	=	= = =	=	= = =	=
{6,4}	t{6,4}	r{6,4}	t{4,6}	{4,6}	rr{6,4}	tr{6,4}
Uniform duals
V64	V4.12.12	V(4.6)2	V6.8.8	V46	V4.4.4.6	V4.8.12
Alternations
[1+,6,4] (*443)	[6+,4] (6*2)	[6,1+,4] (*3222)	[6,4+] (4*3)	[6,4,1+] (*662)	[(6,4,2+)] (2*32)	[6,4]+ (642)
=	=	=	=	=	=
h{6,4}	s{6,4}	hr{6,4}	s{4,6}	h{4,6}	hrr{6,4}	sr{6,4}

Uniform hexahexagonal tilings
Symmetry: [6,6], (*662)
= =	= =	= =	= =	= =	= =	= =
{6,6} = h{4,6}	t{6,6} = h2{4,6}	r{6,6} {6,4}	t{6,6} = h2{4,6}	{6,6} = h{4,6}	rr{6,6} r{6,4}	tr{6,6} t{6,4}
Uniform duals
V66	V6.12.12	V6.6.6.6	V6.12.12	V66	V4.6.4.6	V4.12.12
Alternations
[1+,6,6] (*663)	[6+,6] (6*3)	[6,1+,6] (*3232)	[6,6+] (6*3)	[6,6,1+] (*663)	[(6,6,2+)] (2*33)	[6,6]+ (662)
=		=		=
h{6,6}	s{6,6}	hr{6,6}	s{6,6}	h{6,6}	hrr{6,6}	sr{6,6}

Uniform (4,4,3) tilings
Symmetry: [(4,4,3)] (*443)							[(4,4,3)]+ (443)	[(4,4,3+)] (3*22)	[(4,1+,4,3)] (*3232)
h{6,4} t0(4,4,3)	h2{6,4} t0,1(4,4,3)	{4,6}1/2 t1(4,4,3)	h2{6,4} t1,2(4,4,3)	h{6,4} t2(4,4,3)	r{6,4}1/2 t0,2(4,4,3)	t{4,6}1/2 t0,1,2(4,4,3)	s{4,6}1/2 s(4,4,3)	hr{4,6}1/2 hr(4,3,4)	h{4,6}1/2 h(4,3,4)	q{4,6} h1(4,3,4)
Uniform duals
V(3.4)4	V3.8.4.8	V(4.4)3	V3.8.4.8	V(3.4)4	V4.6.4.6	V6.8.8	V3.3.3.4.3.4	V(4.4.3)2	V66	V4.3.4.6.6

Similar H2 tilings in *3232 symmetry
Coxeter diagrams
Vertex figure	66		(3.4.3.4)2		3.4.6.6.4		6.4.6.4
Image
Dual

References

• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
• "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678. 

See also

Wikimedia Commons has media related to Uniform tiling 3-4-3-4-3-4-3-4.

• Square tiling
• Uniform tilings in hyperbolic plane
• List of regular polytopes

External links

• Weisstein, Eric W. "Hyperbolic tiling". MathWorld. 

• Weisstein, Eric W. "Poincaré hyperbolic disk". MathWorld. 

• Hyperbolic and Spherical Tiling Gallery
• KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
• Hyperbolic Planar Tessellations, Don Hatch

Tessellation
Periodic Pythagorean Rhombille Schwarz triangle Rectangle Domino Uniform tiling & honeycomb Coloring Convex Kisrhombille Wallpaper group Wythoff
Aperiodic Ammann–Beenker Aperiodic set of prototiles List Einstein problem Socolar–Taylor Gilbert Penrose Pentagonal Pinwheel Quaquaversal Rep-tile & Self-tiling Sphinx Truchet
Other Anisohedral & Isohedral Architectonic & catoptric Circle Limit III Computer graphics Honeycomb Isotoxal Packing Problems Domino Wang Heesch's Squaring Prototile Conway criterion Girih Regular Division of the Plane Regular grid Substitution Voronoi Voderberg
By vertex type Spherical 2n 33.n V33.n 42.n V42.n Regular 2∞ 36 44 63 Semi- regular 32.4.3.4 V32.4.3.4 33.42 33.∞ 34.6 V34.6 3.4.6.4 (3.6)2 3.122 42.∞ 4.6.12 4.82 Hyper- bolic 32.4.3.5 32.4.3.6 32.4.3.7 32.4.3.8 32.4.3.∞ 32.5.3.5 32.5.3.6 32.6.3.6 32.6.3.8 32.7.3.7 32.8.3.8 33.4.3.4 32.∞.3.∞ 34.7 34.8 34.∞ 35.4 37 38 3∞ (3.4)3 (3.4)4 3.4.62.4 3.4.7.4 3.4.8.4 3.4.∞.4 3.6.4.6 (3.7)2 (3.8)2 3.142 3.162 (3.∞)2 3.∞2 42.5.4 42.6.4 42.7.4 42.8.4 42.∞.4 45 46 47 48 4∞ (4.5)2 (4.6)2 4.6.12 4.6.14 V4.6.14 4.6.16 V4.6.16 4.6.∞ (4.7)2 (4.8)2 4.8.10 V4.8.10 4.8.12 4.8.14 4.8.16 4.8.∞ 4.102 4.10.12 4.122 4.12.16 4.142 4.162 4.∞2 (4.∞)2 54 55 56 5∞ 5.4.6.4 (5.6)2 5.82 5.102 5.122 (5.∞)2 64 65 66 68 6.4.8.4 (6.8)2 6.82 6.102 6.122 6.162 73 74 77 7.62 7.82 7.142 83 84 86 88 812 8.62 8.162 ∞3 ∞4 ∞5 ∞∞ ∞.62 ∞.82