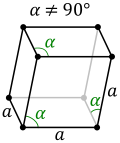
Rhombohedron
| Rhombohedron | |
|---|---|
 | |
| Type | Prism |
| Faces | 6 rhombi |
| Edges | 12 |
| Vertices | 8 |
| Symmetry group | Ci, [2+,2+], (×), Order 2 |
| Properties | convex, zonohedron |
In geometry, a rhombohedron is a three-dimensional figure like a cube, except that its faces are not squares but rhombi. It is a special case of a parallelepiped where all edges are the same length. It can be used to define the rhombohedral lattice system, a honeycomb with rhombohedral cells.
In general the rhombohedron can have three types of rhombic faces in congruent opposite pairs, Ci symmetry, order 2.
Four points forming non-adjacent vertices of a rhombohedron necessarily form the four vertices of an orthocentric tetrahedron, and all orthocentric tetrahedra can be formed in this way.[1]
Rhombohedral lattice system
The rhombohedral lattice system has rhombohedral cells, with 3 pairs of unique rhombic faces:
Special cases
| Form | Cube | Trigonal trapezohedron | Right rhombic prism | General rhombic prism | General rhombohedron |
|---|---|---|---|---|---|
| Symmetry | Oh, [4,3], order 48 | D3d, [2+,6], order 12 | D2h, [2,2], order 8 | C2h, [2], order 4 | Ci, [2+,2+], order 2 |
| Image |  |
 |
 | ||
| Faces | 6 squares | 6 identical rhombi | Two rhombi and 4 squares | 6 rhombic faces | 6 rhombic faces |
- Cube: with Oh symmetry, order 48. All faces are squares.
- Trigonal trapezohedron: with D3d symmetry, order 12. If all of the non-obtuse internal angles of the faces are equal (all faces are same). This can be see by stretching a cube on its body-digonal axis. For example, a regular octahedron with two tetrahedra attached on opposite faces constructs a 60 degree trigonal trapezohedron:.
- Right rhombic prism: with D2h symmetry, order 8. It constructed by two rhombi and 4 squares. This can be see by stretching a cube on its face-digonal axis. For example, two triangular prisms attached together makes a 60 degree rhombic prism.
- A general rhombic prism: With C2h symmetry, order 4. It has only one plane of symmetry through four vertices, and 6 rhombic faces.
Solid Geometry
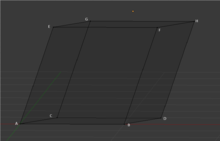
For a unit rhombohedron[2] (side length = 1) whose rhombic acute angle is θ and has one vertex is at the origin (0, 0, 0) with one edge lying along the x-axis the three vectors are
e1:
e2:
e3:
The other coordinates can be obtained from vector addition[3] of the 3 direction vectors so that e1 + e2, e1 + e3, e2 + e3, and e1 + e2 + e3.
The volume of the rhombohedron whose side length is 'a' is a simplification of the volume of a parallelepiped and is given by
As the area of the base is given by and it follows that the height of a rhombohedron is given by the volume divided by the area. The height, 'h', is given by
The internal diagonals of the rhombohedron shown in Fig. SG1 are interesting. Three of the internal diagonals (BG, CF, and DE) are all the same length. They are easily calculated from coordinate geometry once the coordinates of each vertex is known. Distance in a 3-d space is simply given by:[4]
For example, for the unit rhombohedron with rhombic acute angle of 72, the three internal diagonals (BG, CF, and DE) are 1.543 and the long diagonal (AH) is 2.203. The volume of this rhombohedron is 0.8789, and the height is 0.9242.
References
- ↑ Court, N. A. (October 1934), "Notes on the orthocentric tetrahedron", American Mathematical Monthly: 499–502, JSTOR 2300415.
- ↑ Lines, L (1965). Solid geometry: with chapters on space-lattices, sphere-packs and crystals. Dover Publications.
- ↑ "Vector Addition". Wolfram. 17 May 2016. Retrieved 17 May 2016.
- ↑ "Calculate distance in 3D space". Retrieved 17 May 2016.