Plane curve
In mathematics, a plane curve is a curve in a plane that may be either a Euclidean plane, an affine plane or a projective plane. The most frequently studied cases are smooth plane curves (including piecewise smooth plane curves), and algebraic plane curves.
Smooth plane curve
A smooth plane curve is a curve in a real Euclidean plane R2 and is a one-dimensional smooth manifold. This means that a smooth plane curve is a plane curve which "locally looks like a line", in the sense that near every point, it may be mapped to a line by a smooth function. Equivalently, a smooth plane curve can be given locally by an equation f(x, y) = 0, where f : R2 → R is a smooth function, and the partial derivatives ∂f/∂x and ∂f/∂y are never both 0 at a point of the curve.
Algebraic plane curve
An algebraic plane curve is a curve in an affine or projective plane given by one polynomial equation f(x, y) = 0 (or F(x, y, z) = 0, where F is a homogeneous polynomial, in the projective case.)
Algebraic curves were studied extensively since the 18th century.
Every algebraic plane curve has a degree, the degree of the defining equation, which is equal, in case of an algebraically closed field, to the number of intersections of the curve with a line in general position. For example, the circle given by the equation x2 + y2 = 1 has degree 2.
The non-singular plane algebraic curves of degree 2 are called conic sections, and their projective completion are all isomorphic to the projective completion of the circle x2 + y2 = 1 (that is the projective curve of equation x2 + y2 - z2= 0). The plane curves of degree 3 are called cubic plane curves and, if they are non-singular, elliptic curves. Those of degree four are called quartic plane curves.
Examples
| Name | Implicit equation | Parametric equation | As a function | graph |
|---|---|---|---|---|
| Straight line | 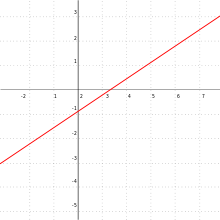 | |||
| Circle |  | |||
| Parabola | 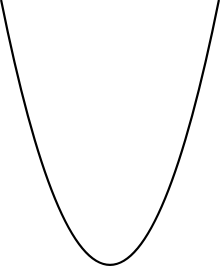 | |||
| Ellipse |  | |||
| Hyperbola | 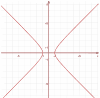 |
See also
- Algebraic curve
- Differential geometry
- Algebraic geometry
- Plane curve fitting
- Projective varieties
- Two-dimensional graph
References
- Coolidge, J. L. (April 28, 2004), A Treatise on Algebraic Plane Curves, Dover Publications, ISBN 0-486-49576-0.
- Yates, R. C. (1952), A handbook on curves and their properties, J.W. Edwards, ASIN B0007EKXV0.