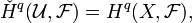Leray's theorem

In algebraic geometry, Leray's theorem relates abstract sheaf cohomology with Čech cohomology.

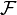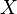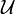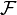Let $\mathcal F$ be a sheaf on a topological space $X$ and $\mathcal U$ an open cover of $X.$ If $\mathcal F$ is acyclic on every finite intersection of elements of 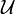$\mathcal U$ , then

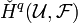\check H^q(\mathcal U,\mathcal F)= H^q(X,\mathcal F),

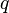where $\check H^q(\mathcal U,\mathcal F)$ is the $q$ -th Čech cohomology group of 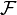$\mathcal F$ with respect to the open cover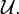 $\mathcal U.$

References

Bonavero, Laurent. Cohomology of Line Bundles on Toric Varieties, Vanishing Theorems. Lectures 16-17 from "Summer School 2000: Geometry of Toric Varieties."

This article incorporates material from Leray's theorem on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.

This article is issued from Wikipedia - version of the 2/17/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.