Spherical cap

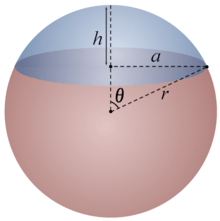
An example of a spherical cap in blue.

In geometry, a spherical cap, spherical dome, or spherical segment of one base is a portion of a sphere cut off by a plane. If the plane passes through the center of the sphere, so that the height of the cap is equal to the radius of the sphere, the spherical cap is called a hemisphere.

Volume and surface area

If the radius of the base of the cap is $a$ , and the height of the cap is $h$ , then the volume of the spherical cap is^[1]

V={\frac {\pi h}{6}}(3a^{2}+h^{2})

and the curved surface area of the spherical cap is^[1]

A=2\pi rh

A=2\pi r^{2}(1-\cos \theta )

The relationship between $h$ and $r$ is irrelevant as long as $0\leq h\leq 2r$ . The red section of the illustration is also a spherical cap.

The parameters $a$ , $h$ and $r$ are not independent:

r^{2}=(r-h)^{2}+a^{2}=r^{2}+h^{2}-2rh+a^{2}

r={\frac {a^{2}+h^{2}}{2h}}

Substituting this into the area formula gives:

A=2\pi {\frac {(a^{2}+h^{2})}{2h}}h=\pi (a^{2}+h^{2})

Note also that in the upper hemisphere of the diagram, $h=r-{\sqrt {r^{2}-a^{2}}}$ , and in the lower hemisphere $h=r+{\sqrt {r^{2}-a^{2}}}$ ; hence in either hemisphere $a={\sqrt {h(2r-h)}}$ and so an alternative expression for the volume is

V={\frac {\pi h^{2}}{3}}(3r-h)

The volume may also be found by integrating under a surface of rotation, using $\scriptstyle x=r\cos(\theta )$ and factorizing.

V=\int _{x}^{r}\pi \left(r^{2}-x^{2}\right)dx=\pi \left({\frac {2}{3}}r^{3}-r^{2}x+{\frac {1}{3}}x^{3}\right)={\frac {\pi }{3}}r^{3}(\cos(\theta )+2)(\cos(\theta )-1)^{2}

Applications

Volumes of union and intersection of two intersecting spheres

The volume of the union of two intersecting spheres of radii $r 1$ and $r 2$ is ^[2]

V=V^{(1)}-V^{(2)}

where

V^{(1)}={\frac {4\pi }{3}}r_{1}^{3}+{\frac {4\pi }{3}}r_{2}^{3}

is the sum of the volumes of the two isolated spheres, and

V^{(2)}={\frac {\pi h_{1}^{2}}{3}}(3r_{1}-h_{1})+{\frac {\pi h_{2}^{2}}{3}}(3r_{2}-h_{2})

the sum of the volumes of the two spherical caps forming their intersection. If $d < r 1 + r 2$ is the distance between the two sphere centers, elimination of the variables $h 1$ and $h 2$ leads to^[3]^[4]

V^{(2)}={\frac {\pi }{12d}}(r_{1}+r_{2}-d)^{2}\left(d^{2}+2d(r_{1}+r_{2})-3(r_{1}-r_{2})^{2}\right)

Surface area bounded by circles of latitude

The surface area bounded by two circles of latitude is the difference of surface areas of their respective spherical caps. For a sphere of radius r, and latitudes φ₁ and φ₂, the area is ^[5]

A=2\pi r^{2}|\sin \phi _{1}-\sin \phi _{2}|

For example, assuming the Earth is a sphere of radius 6371 km, the surface area of the arctic (north of the Arctic Circle, at latitude 66.56° as of August 2016^[6]) is 2 $π$ ·6371²|sin 90° − sin 66.56°| = 21.04 million km², or 0.5·|sin 90° − sin 66.56°| = 4.125% of the total surface area of the Earth.

Generalizations

Sections of other solids

The spheroidal dome is obtained by sectioning off a portion of a spheroid so that the resulting dome is circularly symmetric (having an axis of rotation), and likewise the ellipsoidal dome is derived from the ellipsoid.

Hyperspherical cap

Generally, the $n$ -dimensional volume of a hyperspherical cap of height $h$ and radius $r$ in $n$ -dimensional Euclidean space is given by ^[7]

V={\frac {\pi ^{\frac {n-1}{2}}\,r^{n}}{\,\Gamma \left({\frac {n+1}{2}}\right)}}\int \limits _{0}^{\arccos \left({\frac {r-h}{r}}\right)}\sin ^{n}(t)\,\mathrm {d} t

where $\Gamma$ (the gamma function) is given by $\Gamma (z)=\int _{0}^{\infty }t^{z-1}\mathrm {e} ^{-t}\,\mathrm {d} t$ .

The formula for $V$ can be expressed in terms of the volume of the unit n-ball $C_{n}={\scriptstyle \pi ^{n/2}/\Gamma [1+{\frac {n}{2}}]}$ and the hypergeometric function ${}_{2}F_{1}$ or the regularized incomplete beta function $I_{x}(a,b)$ as

V=C_{n}\,r^{n}\left({\frac {1}{2}}\,-\,{\frac {r-h}{r}}\,{\frac {\Gamma [1+{\frac {n}{2}}]}{{\sqrt {\pi }}\,\Gamma [{\frac {n+1}{2}}]}}{\,\,}_{2}F_{1}\left({\tfrac {1}{2}},{\tfrac {1-n}{2}};{\tfrac {3}{2}};\left({\tfrac {r-h}{r}}\right)^{2}\right)\right)={\frac {1}{2}}C_{n}\,r^{n}I_{(2rh-h^{2})/r^{2}}\left({\frac {n+1}{2}},{\frac {1}{2}}\right)

and the area formula $A$ can be expressed in terms of the area of the unit n-ball $A_{n}={\scriptstyle 2\pi ^{n/2}/\Gamma [{\frac {n}{2}}]}$ as

A={\frac {1}{2}}A_{n}\,r^{n-1}I_{(2rh-h^{2})/r^{2}}\left({\frac {n-1}{2}},{\frac {1}{2}}\right)

where $\scriptstyle 0\leq h\leq r$ .

Earlier in ^[8] (1986, USSR Academ. Press) the following formulas were derived: $A=A_{n}p_{n-2}(q),V=C_{n}p_{n}(q)$ , where $q=1-h/r(0\leq q\leq 1),p_{n}(q)=(1-G_{n}(q)/G_{n}(1))/2$ ,

$G_{n}(q)=\int \limits _{0}^{q}(1-t^{2})^{(n-1)/2}dt$ .

For odd $n=2k+1:$

$G_{n}(q)=\sum _{i=0}^{k}(-1)^{i}{\binom {k}{i}}{\frac {q^{2i+1}}{2i+1}}$ .

It is shown in ^[9] that, if $n\to \infty$ and $q{\sqrt {n}}={\text{const.}}$ , then $p_{n}(q)\to 1-F({q{\sqrt {n}}})$ where $F()$ is the integral of the standard normal distribution.

References

1 2 Polyanin, Andrei D; Manzhirov, Alexander V. (2006), Handbook of Mathematics for Engineers and Scientists, CRC Press, p. 69, ISBN 9781584885023 .
↑ Connolly, Michael L. (1985). "Computation of molecular volume". J. Am. Chem. Soc. 107: 1118–1124. doi:10.1021/ja00291a006.
↑ Pavani, R.; Ranghino, G. (1982). "A method to compute the volume of a molecule". Comput. Chem. 6: 133–135. doi:10.1016/0097-8485(82)80006-5.
↑ Bondi, A. (1964). "Van der Waals volumes and radii". J. Phys. Chem. 68: 441–451. doi:10.1021/j100785a001.
↑ Scott E. Donaldson, Stanley G. Siegel. "Successful Software Development". Retrieved 29 August 2016.
↑ "Obliquity of the Ecliptic (Eps Mean)". Neoprogrammics.com. Retrieved 2014-05-13.
↑ Li, S (2011). "Concise Formulas for the Area and Volume of a Hyperspherical Cap". Asian J. Math. Stat. 4 (1): 66–70. doi:10.3923/ajms.2011.66.70.
↑ Chudnov, Alexander M. (1986). "On minimax signal generation and reception algorithms (rus.)". Problems of Information Transmission. 22 (4): 49–54.
↑ Chudnov, Alexander M (1991). "Game-theoretical problems of synthesis of signal generation and reception algorithms (rus.)". Problems of Information Transmission. 27 (3): 57–65.

Additional reading

Richmond, Timothy J. (1984). "Solvent accessible surface area and excluded volume in proteins: Analytical equation for overlapping spheres and implications for the hydrophobic effect". J. Mol. Biol. 178 (1): 63–89. doi:10.1016/0022-2836(84)90231-6.
Lustig, Rolf (1986). "Geometry of four hard fused spheres in an arbitrary spatial configuration". Mol. Phys. 59 (2): 195–207. Bibcode:1986MolPh..59..195L. doi:10.1080/00268978600102011.
Gibson, K. D.; Scheraga, Harold A. (1987). "Volume of the intersection of three spheres of unequal size: a simplified formula". J. Phys. Chem. 91 (15): 4121–4122. doi:10.1021/j100299a035.
Gibson, K. D.; Scheraga, Harold A. (1987). "Exact calculation of the volume and surface area of fused hard-sphere molecules with unequal atomic radii". Mol. Phys. 62 (5): 1247–1265. Bibcode:1987MolPh..62.1247G. doi:10.1080/00268978700102951.
Petitjean, Michel (1994). "On the analytical calculation of van der Waals surfaces and volumes: some numerical aspects". Int. J. Quant. Chem. 15 (5): 507–523. doi:10.1002/jcc.540150504.
Grant, J. A.; Pickup, B. T. (1995). "A Gaussian description of molecular shape". J. Phys. Chem. 99 (11): 3503–3510. doi:10.1021/j100011a016.
Busa, Jan; Dzurina, Jozef; Hayryan, Edik; Hayryan, Shura (2005). "ARVO: A fortran package for computing the solvent accessible surface area and the excluded volume of overlapping spheres via analytic equations". Comp. Phys. Commun. 165: 59–96. Bibcode:2005CoPhC.165...59B. doi:10.1016/j.cpc.2004.08.002.

External links

Wikimedia Commons has media related to Spherical caps.

Weisstein, Eric W. "Spherical cap". MathWorld.

Derivation and some additional formulas.

This article is issued from Wikipedia - version of the 11/4/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.