Boundary-incompressible surface

In low-dimensional topology, a boundary-incompressible surface is a two-dimensional surface within a three-dimensional manifold whose topology cannot be made simpler by a certain type of operation known as boundary compression.

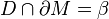Suppose M is a 3-manifold with boundary. Suppose also that S is a compact surface with boundary that is properly embedded in M, meaning that the boundary of S is a subset of the boundary of M and the interior points of S are a subset of the interior points of M. 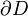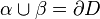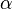A boundary-compressing disk for S in M is defined to be a disk D in M such that $D \cap S = \alpha$ and $D \cap \partial M = \beta$ are arcs in $\partial D$ , with $\alpha \cup \beta = \partial D$ , $\alpha \cap \beta = \partial \alpha = \partial \beta$ , and $\alpha$ is an essential arc in S ( 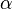$\alpha$ does not cobound a disk in S with another arc in $\partial S$ ).

The surface S is said to be boundary-compressible if either S is a disk that cobounds a ball with a disk in 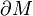$\partial M$ or there exists a boundary-compressing disk for S in M. Otherwise, S is boundary-incompressible.

Alternatively, one can relax this definition by dropping the requirement that the surface be properly embedded. Suppose now that S is a compact surface (with boundary) embedded in the boundary of a 3-manifold M. Suppose further that D is a properly embedded disk in M such that D intersects S in an essential arc (one that does not cobound a disk in S with another arc in $\partial S$ ). Then D is called a boundary-compressing disk for S in M. 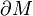As above, S is said to be boundary-compressible if either S is a disk in $\partial M$ or there exists a boundary-compressing disk for S in M. Otherwise, S is boundary-incompressible.

For instance, if K is a trefoil knot embedded in the boundary of a solid torus V and S is the closure of a small annular neighborhood of K in $\partial V$ , then S is not properly embedded in V since the interior of S is not contained in the interior of V. However, S is embedded in $\partial V$ and there does not exist a boundary-compressing disk for S in V, so S is boundary-incompressible by the second definition.

References

W. Jaco, Lectures on Three-Manifold Topology, volume 43 of CBMS Regional Conference Series in Mathematics. American Mathematical Society, Providence, R.I., 1980.
T. Kobayashi, A construction of 3-manifolds whose homeomorphism classes of Heegaard splittings have polynomial growth, Osaka J. Math. 29 (1992), no. 4, 653–674. MR 93j:57007.

This article is issued from Wikipedia - version of the 4/1/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Boundary-incompressible surface

See also

References